《線性混合整數優化》(Gurobi Optimization Gurobi)v4.0.1[壓縮包] 簡介: 中文名 : 線性混合整數優化 英文名 : Gurobi Optimization Gurobi 資源格式 : 壓縮包 版本 : v4.0.1 發行時間 : 2010年 制作發行 : Gurobi Optimization 地區 : 美國 語言 : 英文 簡介 : 軟件類型:行業軟件-整數優化 軟件性質:破解軟件 操作系統:Windows 應用平台:Winll 問題
電驢資源下載/磁力鏈接資源下載:
全選
"《線性混合整數優化》(Gurobi Optimization Gurobi)v4.0.1[壓縮包]"介紹
中文名: 線性混合整數優化
英文名: Gurobi Optimization Gurobi
資源格式: 壓縮包
版本: v4.0.1
發行時間: 2010年
制作發行: Gurobi Optimization
地區: 美國
語言: 英文
簡介:

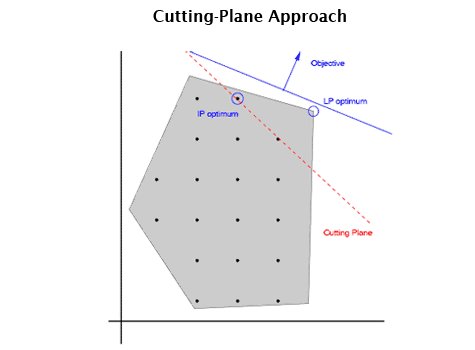
軟件類型:行業軟件-整數優化
軟件性質:破解軟件
操作系統:Windows
應用平台:Winll
問題反饋: http://www.gurobi.com/html/contact.html
網站鏈接: http://www.gurobi.com/
軟件介紹:
Gurobi 4 隆重發布,在數學優化器領域繼續擴大領先優勢。主要特色包括:
新增 QP 和 MIQP 優化器;
在版本3基礎上,線性和混合整數問題求解速度進一步提升;
數值計算穩定性進一步提升;
並發 LP 計算;
新增 MIP 終止計算策略選項;
支持和 Visual Studio 2010 集成
Java 和 .Net 環境下浮動許可的更多自主控制。
Gurobi 特點
Gurobi 具有許多獨特的特點和功能,可以使得用戶迅速而准確地獲得最優結果。這些特點包括:
采用最新優化技術,充分利用多核處理器優勢
任何版本都支持並行計算,並且計算結果確定而非隨機
提供了方便輕巧的接口,支持 C++, Java, Python, .Net 開發,內存消耗少
支持多種平台,包括 Windows, Linux, Mac OS X
支持 AMPL、GAMS、AIMMS和 Windows Solver Foundation 建模環境
單一版本,開發版本也就是發布版本,程序轉移便捷
性價比突出,為學校、企業提供了差異化價格,方便各種需求
第三方商業和免費軟件支持和Matlab接口
強大的技術支持力量,Gurobi 提供中英文雙語技術支持
完備的用戶使用手冊
Gurobi 可以解決的問題
Gurobi 可以解決的數學問題:
線性問題(Linear problems)
二次型目標問題(Quadratic problems)
混合整數線性和二次型問題(Mixed integer linear and quadratic problems)
突出的性價比
Gurobi 不區分開發許可和實施許可,一個許可軟件既可以用在開發上也可以用在實施上。同時,允許一個許可軟件應用於多個應用程序上,極大地降低了大型優化項目的開發和實施成本。
應用領域
線性混合整數優化是應用在各個領域中最常見的優化方法之一,是過去30年當中在實際應用中創造價值最巨大的優化方法。在物流、生產制造、金融、交通運輸、資源管理、集成電路設計、環境保護、電力管理等等領域,幾乎無所不在。在世界一流的企業資源管理(ERP)、供應鏈管理(SCM)、運輸管理等企業決策工具中,都有線性混合整數優化器的存在。
The Gurobi Optimizer
The Gurobi Optimizer is a state-of-the-art solver for linear programming (LP), quadratic programming (QP) and mixed-integer programming (MILP and MIQP). It was designed from the ground up to exploit modern multi-core processors.
For solving LP and QP models, the Gurobi Optimizer includes high-performance implementations of the primal simplex method, the dual simplex method, and a parallel barrier solver. For MILP and MIQP models, the Gurobi Optimizer incorporates the latest methods including cutting planes and powerful solution heuristics. All models benefit from advanced presolve methods to simplify models and slash solve times.
Every Gurobi license allows parallel processing, and the Gurobi Parallel Optimizer is deterministic: two separate runs on the same model will produce identical solution paths.
The Gurobi Optimizer is written in C and is accessible from several languages. In addition to a powerful, interactive Python interface and a matrix-oriented C interface, we provide object-oriented interfaces from C++, Java, Python, and the .NET languages. These interfaces have all been designed to be lightweight and easy to use, with the goal of greatly enhancing the accessibility of our products. And since the interfaces are lightweight, they are faster and use less memory than other standard interfaces. Our online documentation (Quick Start Guide, Example Tour and Reference Manual) describes the use of these interfaces.
The Gurobi Optimizer is available for popular computing platforms including Microsoft Windows, Linux and Mac OS X; a full list is available in the Platforms table.
Accessing the Gurobi Optimizer
We offer commercial licenses that support a variety of usage scenarios. You can purchase licenses for a single system, floating licenses that allow several users on a network to use Gurobi, and licenses for embedding Gurobi inside your product. Pricing information can be found in our client area; please Login and select 'Pricing' on the left for additional information. To purchase a license, please click here for contact information.
A free Trial version is available for immediate download and installation. This version will accept problems with up to 500 variables and 500 constraints. With the exception of these size restrictions, this version is full featured, including access to all Gurobi solvers and interfaces.
We also offer free Academic Licenses to faculty, students, and staff at qualifying academic institutions. These licenses have no size restrictions. They provide complete access to the full set of features of our commercial product.
We also offer Gurobi Cloud, which allows you to use the Gurobi Optimizer on an hourly basis via the Amazon Elastic Computing Cloud (EC2).
The Gurobi solvers are also available through a number of powerful third-party modeling systems. Gurobi Optimization is an authorized reseller for two of these systems: AMPL and Microsoft Solver Foundation. For AMPL, please Login to the Gurobi client area and select 'Pricing' on the left for additional information on purchasing this systems through us. For more information about Microsoft Solver Foundation, please send email to info[at]gurobi.com.
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
更多資訊請點擊訪問

||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
本資源下載鏈接來自ShareVirus
代碼
± SINCE 2000 ±
E A T P R E S E N T S
Gurobi.Optimization.Gurobi.v4.0.1.Cracked-EAT
±²² ²²±
²²°² RELEASE INFO ²°²²
° ± ± °
° ± SUPPLIER ....: TEAM EAT ± °
± PROG TYPE ...: SCIENTIFIC ±
° LANGUAGE ....: ENGLISH °
RELEASE DATE.: 2011-01-28
° °
° CRACKER ......: TEAM EAT °
PROTECTION ...: DEMO-LIMITS
DIFFICULTY ...: GUESS!
PACKAGER ....: TEAM EAT
FORMAT ......: ZIP/RAR
ARCHIVE NAME.: eatgb40a.zip
No OF DISKS .: [XX/02]
REQUIREMENTS .: WinXP/Vista/Win7
PRICE ........: $8,500.00
WEBSITE.......: http://www.gurobi.com
²²°² RELEASE NOTES ²°²²
The Gurobi Optimizer is a state-of-the-art solver
for linear programming (LP), quadratic programming
(QP) and mixed-integer programming (MILP and MIQP).
It was designed from the ground up to exploit modern
multi-core processors. Every Gurobi license allows
parallel processing, and the Gurobi Parallel
Optimizer is deterministic: two separate runs on the
same model will produce identical solution paths.
For solving LP and QP models, the Gurobi Optimizer
includes high-performance implementations of the
primal simplex method, the dual simplex method, and
a parallel barrier solver. For MILP and MIQP models,
the Gurobi Optimizer incorporates the latest methods
including cutting planes and powerful solution
heuristics. All models benefit from advanced
presolve methods to simplify models and slash solve
times.
The Gurobi Optimizer is written in C and is
accessible from several languages. In addition to a
powerful, interactive Python interface and a
matrix-oriented C interface, we provide
object-oriented interfaces from C++, Java, Python,
and the .NET languages. These interfaces have all
been designed to be lightweight and easy to use,
with the goal of greatly enhancing the accessibility
of our products. And since the interfaces are
lightweight, they are faster and use less memory
than other standard interfaces. Our online
documentation (Quick Start Guide, Example Tour and
Reference Manual) describes the use of these
interfaces.
Gurobi is also available through several powerful
third-party modeling systems including AIMMS, AMPL,
FRONTLINE SOLVERS, GAMS, MPL, OptimJ and TOMLAB.
Version 4.0 of the Gurobi Optimizer includes a
number of enhancements. Users of previous versions
may need to make a few minor changes to their
existing programs. Here are the new features, and
the likely changes required to existing programs.
New features:
* Quadratic programming: The Gurobi Optimizer now
supports models with quadratic objective
functions. The new version includes primal
simplex, dual simplex, and parallel barrier
optimizers for continuous QP models, and a
parallel branch-and-cut solver for Mixed Integer
Quadratic Programming (MIQP) models.
* Concurrent optimizer: The Gurobi Optimizer now
allows you to run multiple algorithms
simultaneously when solving a linear continuous
model on a multi-core machine. The optimizer
returns when the first algorithm solves the model.
We include both a standard concurrent optimizer
and a deterministic concurrent optimizer. The
latter returns the exact same solution every time
you run it, while the former can sometimes return
different optimal solutions from one run to the
next. The former can sometimes be significantly
faster.
* MIP performance: The MIP solver is faster in
release 4.0. These improvements do not require any
parameter changes.
* LP performance: The simplex and barrier solvers
are slightly faster in release 4.0. We have also
improved the numerical stability of the primal
simplex solver and the barrier crossover
algorithm.
* Delayed MIP strategy change: The Gurobi Optimizer
now gives you the option to change a few MIP
parameters in the middle of the optimization in
order to dynamically shift the search strategy.
Specifically, two new parameters, ImproveStartGap
and ImproveStartTime, allow you to specify when
the algorithm should modify the values of a few
parameters that control the intensity of the MIP
heuristics. By setting one or both of these
parameters to non-default values, you can cause
the MIP solver to switch from its standard
parameter settings, where it tries to strike a
balance between finding better solutions and
proving that the current solution is optimal, to a
set of parameter values that focus almost entirely
on finding better solutions.
* Support for Visual Studio 2010: Gurobi Optimizer
now supports Microsoft Visual Studio 2010. This
change only affects C++ users, who will find new
libraries gurobi_c++2010md.lib,
gurobi_c++2010mdd.lib, gurobi_c++2010mt.lib, and
gurobi_c++2010mtd.lib in the lib directory of the
Gurobi distribution.
* Explicit license release in Java and .NET: The new
version includes an explicit method for releasing
a Gurobi license. You no longer need to rely on
the garbage collector to reclaim unused licenses.
* New methods, attributes, parameters, and error
codes: To support the new features in Gurobi 4.0,
we have added several new methods, attributes,
parameters, and error codes. You can learn more
about these in the {Gurobi Reference Manual}.
New methods:
* New C methods for managing Q: the C interface
includes three new routines, GRBaddqpterms,
GRBdelq, and GRBgetq. These allow you to add,
delete, and retrieve quadratic objective terms,
respectively.
* Quadratic expressions: the object oriented
interfaces include a new quadratic expression
class, GRBQuadExpr, which can be used to build
quadratic objective functions.
* getObjective/setObjective: the object oriented
interfaces include new GRBModel methods that allow
you to retrieve the current objective function as
a linear or quadratic expression, and allow you to
set the objective equal to a linear or quadratic
expression.
* getValue: the object oriented interfaces include a
new getValue method that allows you to compute the
value of a GRBLinExpr or GRBQuadExpr object for
the current solution.
* License release: the Java and .NET interfaces
include a new release method that allows you to
release the license held by an environment
immediately, instead of having to wait for the
garbage collector to reclaim the GRBEnv object.
New attributes:
* IsQP: model attribute that indicates whether the
model has any quadratic terms.
* NumQNZs: model attribute that returns the number
of quadratic terms in the objective function.
New parameters:
* Method: the previous LPMethod parameter has been
renamed to Method. This new parameter controls the
algorithm used to solve continuous linear and
quadratic models. We have added two new options:
concurrent and deterministic concurrent. This
parameter also selects the algorithm used to solve
the root node of a MIP model.
* NodeMethod: chooses the algorithm used to solve
node relaxations in a MIP model.
* ModKCuts: controls the generation of mod-k cuts.
* ImproveStartGap: allows you to specify the
optimality gap at which the MIP solver resets a
few MIP heuristics parameters in order to shift
the attention of the MIP solver to finding the
best possible feasible solution.
* ImproveStartTime: allows you to specify the
elapsed time at which the MIP solver resets a few
MIP heuristics parameters in order to shift the
attention of the MIP solver to finding the best
possible feasible solution.
* PreMIQPMethod: chooses the presolve transformation
performed on MIQP models.
* PSDTol: sets a limit on the amount of diagonal
perturbation that the optimizer is allowed to do
on the Q matrix for a quadratic model. If a larger
perturbation is required, the optimizer will
terminate with an GRB_ERROR_Q_NOT_PSD error.
New error codes:
* GRB_ERROR_Q_NOT_PSD: This new error code is
returned when you attempt to solve a QP model
where the Q matrix is not positive semi-definite
(meaning there exists an x for which x'Qx ). Note
that the optimizer will always try to add a small
perturbation to the diagonal to correct small PSD
violations. This error will be reported when the
required perturbation is too large (as controlled
by the new PSDTol parameter).
° ²° COMMENTS °² °
Do NOT distribute this release outside of the scene
Keep the scene alive and secure!
All good progs start as freeware,
then things get worse ...;-)
±²² ²²±
²²°² INSTALLATION NOTES ²°²²
±² ² `TLB' ² ²±
± ± Try it, Like it, Buy it! ± ±
° ° °
1. Unpack and install.
2. Copy the included files over the originals.
That's all. Have fun using it!;-)
___________________________________________________________________
Always remember to block applications (or go off line) from calling
home 'during install'. Once installed, disable 'check for automatic
updates' option if available, so that you don't get it blacklisted.

本資源帖謝絕任何廣告及與資源無關的評論,凡涉及廣告內容的評論一概刪除處理!
英文名: Gurobi Optimization Gurobi
資源格式: 壓縮包
版本: v4.0.1
發行時間: 2010年
制作發行: Gurobi Optimization
地區: 美國
語言: 英文
簡介:


軟件類型:行業軟件-整數優化
軟件性質:破解軟件
操作系統:Windows
應用平台:Winll
問題反饋: http://www.gurobi.com/html/contact.html
網站鏈接: http://www.gurobi.com/
軟件介紹:
Gurobi 4 隆重發布,在數學優化器領域繼續擴大領先優勢。主要特色包括:
新增 QP 和 MIQP 優化器;
在版本3基礎上,線性和混合整數問題求解速度進一步提升;
數值計算穩定性進一步提升;
並發 LP 計算;
新增 MIP 終止計算策略選項;
支持和 Visual Studio 2010 集成
Java 和 .Net 環境下浮動許可的更多自主控制。
Gurobi 特點
Gurobi 具有許多獨特的特點和功能,可以使得用戶迅速而准確地獲得最優結果。這些特點包括:
采用最新優化技術,充分利用多核處理器優勢
任何版本都支持並行計算,並且計算結果確定而非隨機
提供了方便輕巧的接口,支持 C++, Java, Python, .Net 開發,內存消耗少
支持多種平台,包括 Windows, Linux, Mac OS X
支持 AMPL、GAMS、AIMMS和 Windows Solver Foundation 建模環境
單一版本,開發版本也就是發布版本,程序轉移便捷
性價比突出,為學校、企業提供了差異化價格,方便各種需求
第三方商業和免費軟件支持和Matlab接口
強大的技術支持力量,Gurobi 提供中英文雙語技術支持
完備的用戶使用手冊
Gurobi 可以解決的問題
Gurobi 可以解決的數學問題:
線性問題(Linear problems)
二次型目標問題(Quadratic problems)
混合整數線性和二次型問題(Mixed integer linear and quadratic problems)
突出的性價比
Gurobi 不區分開發許可和實施許可,一個許可軟件既可以用在開發上也可以用在實施上。同時,允許一個許可軟件應用於多個應用程序上,極大地降低了大型優化項目的開發和實施成本。
應用領域
線性混合整數優化是應用在各個領域中最常見的優化方法之一,是過去30年當中在實際應用中創造價值最巨大的優化方法。在物流、生產制造、金融、交通運輸、資源管理、集成電路設計、環境保護、電力管理等等領域,幾乎無所不在。在世界一流的企業資源管理(ERP)、供應鏈管理(SCM)、運輸管理等企業決策工具中,都有線性混合整數優化器的存在。
The Gurobi Optimizer
The Gurobi Optimizer is a state-of-the-art solver for linear programming (LP), quadratic programming (QP) and mixed-integer programming (MILP and MIQP). It was designed from the ground up to exploit modern multi-core processors.
For solving LP and QP models, the Gurobi Optimizer includes high-performance implementations of the primal simplex method, the dual simplex method, and a parallel barrier solver. For MILP and MIQP models, the Gurobi Optimizer incorporates the latest methods including cutting planes and powerful solution heuristics. All models benefit from advanced presolve methods to simplify models and slash solve times.
Every Gurobi license allows parallel processing, and the Gurobi Parallel Optimizer is deterministic: two separate runs on the same model will produce identical solution paths.
The Gurobi Optimizer is written in C and is accessible from several languages. In addition to a powerful, interactive Python interface and a matrix-oriented C interface, we provide object-oriented interfaces from C++, Java, Python, and the .NET languages. These interfaces have all been designed to be lightweight and easy to use, with the goal of greatly enhancing the accessibility of our products. And since the interfaces are lightweight, they are faster and use less memory than other standard interfaces. Our online documentation (Quick Start Guide, Example Tour and Reference Manual) describes the use of these interfaces.
The Gurobi Optimizer is available for popular computing platforms including Microsoft Windows, Linux and Mac OS X; a full list is available in the Platforms table.
Accessing the Gurobi Optimizer
We offer commercial licenses that support a variety of usage scenarios. You can purchase licenses for a single system, floating licenses that allow several users on a network to use Gurobi, and licenses for embedding Gurobi inside your product. Pricing information can be found in our client area; please Login and select 'Pricing' on the left for additional information. To purchase a license, please click here for contact information.
A free Trial version is available for immediate download and installation. This version will accept problems with up to 500 variables and 500 constraints. With the exception of these size restrictions, this version is full featured, including access to all Gurobi solvers and interfaces.
We also offer free Academic Licenses to faculty, students, and staff at qualifying academic institutions. These licenses have no size restrictions. They provide complete access to the full set of features of our commercial product.
We also offer Gurobi Cloud, which allows you to use the Gurobi Optimizer on an hourly basis via the Amazon Elastic Computing Cloud (EC2).
The Gurobi solvers are also available through a number of powerful third-party modeling systems. Gurobi Optimization is an authorized reseller for two of these systems: AMPL and Microsoft Solver Foundation. For AMPL, please Login to the Gurobi client area and select 'Pricing' on the left for additional information on purchasing this systems through us. For more information about Microsoft Solver Foundation, please send email to info[at]gurobi.com.
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
更多資訊請點擊訪問

||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
本資源下載鏈接來自ShareVirus
代碼
± SINCE 2000 ±
E A T P R E S E N T S
Gurobi.Optimization.Gurobi.v4.0.1.Cracked-EAT
±²² ²²±
²²°² RELEASE INFO ²°²²
° ± ± °
° ± SUPPLIER ....: TEAM EAT ± °
± PROG TYPE ...: SCIENTIFIC ±
° LANGUAGE ....: ENGLISH °
RELEASE DATE.: 2011-01-28
° °
° CRACKER ......: TEAM EAT °
PROTECTION ...: DEMO-LIMITS
DIFFICULTY ...: GUESS!
PACKAGER ....: TEAM EAT
FORMAT ......: ZIP/RAR
ARCHIVE NAME.: eatgb40a.zip
No OF DISKS .: [XX/02]
REQUIREMENTS .: WinXP/Vista/Win7
PRICE ........: $8,500.00
WEBSITE.......: http://www.gurobi.com
²²°² RELEASE NOTES ²°²²
The Gurobi Optimizer is a state-of-the-art solver
for linear programming (LP), quadratic programming
(QP) and mixed-integer programming (MILP and MIQP).
It was designed from the ground up to exploit modern
multi-core processors. Every Gurobi license allows
parallel processing, and the Gurobi Parallel
Optimizer is deterministic: two separate runs on the
same model will produce identical solution paths.
For solving LP and QP models, the Gurobi Optimizer
includes high-performance implementations of the
primal simplex method, the dual simplex method, and
a parallel barrier solver. For MILP and MIQP models,
the Gurobi Optimizer incorporates the latest methods
including cutting planes and powerful solution
heuristics. All models benefit from advanced
presolve methods to simplify models and slash solve
times.
The Gurobi Optimizer is written in C and is
accessible from several languages. In addition to a
powerful, interactive Python interface and a
matrix-oriented C interface, we provide
object-oriented interfaces from C++, Java, Python,
and the .NET languages. These interfaces have all
been designed to be lightweight and easy to use,
with the goal of greatly enhancing the accessibility
of our products. And since the interfaces are
lightweight, they are faster and use less memory
than other standard interfaces. Our online
documentation (Quick Start Guide, Example Tour and
Reference Manual) describes the use of these
interfaces.
Gurobi is also available through several powerful
third-party modeling systems including AIMMS, AMPL,
FRONTLINE SOLVERS, GAMS, MPL, OptimJ and TOMLAB.
Version 4.0 of the Gurobi Optimizer includes a
number of enhancements. Users of previous versions
may need to make a few minor changes to their
existing programs. Here are the new features, and
the likely changes required to existing programs.
New features:
* Quadratic programming: The Gurobi Optimizer now
supports models with quadratic objective
functions. The new version includes primal
simplex, dual simplex, and parallel barrier
optimizers for continuous QP models, and a
parallel branch-and-cut solver for Mixed Integer
Quadratic Programming (MIQP) models.
* Concurrent optimizer: The Gurobi Optimizer now
allows you to run multiple algorithms
simultaneously when solving a linear continuous
model on a multi-core machine. The optimizer
returns when the first algorithm solves the model.
We include both a standard concurrent optimizer
and a deterministic concurrent optimizer. The
latter returns the exact same solution every time
you run it, while the former can sometimes return
different optimal solutions from one run to the
next. The former can sometimes be significantly
faster.
* MIP performance: The MIP solver is faster in
release 4.0. These improvements do not require any
parameter changes.
* LP performance: The simplex and barrier solvers
are slightly faster in release 4.0. We have also
improved the numerical stability of the primal
simplex solver and the barrier crossover
algorithm.
* Delayed MIP strategy change: The Gurobi Optimizer
now gives you the option to change a few MIP
parameters in the middle of the optimization in
order to dynamically shift the search strategy.
Specifically, two new parameters, ImproveStartGap
and ImproveStartTime, allow you to specify when
the algorithm should modify the values of a few
parameters that control the intensity of the MIP
heuristics. By setting one or both of these
parameters to non-default values, you can cause
the MIP solver to switch from its standard
parameter settings, where it tries to strike a
balance between finding better solutions and
proving that the current solution is optimal, to a
set of parameter values that focus almost entirely
on finding better solutions.
* Support for Visual Studio 2010: Gurobi Optimizer
now supports Microsoft Visual Studio 2010. This
change only affects C++ users, who will find new
libraries gurobi_c++2010md.lib,
gurobi_c++2010mdd.lib, gurobi_c++2010mt.lib, and
gurobi_c++2010mtd.lib in the lib directory of the
Gurobi distribution.
* Explicit license release in Java and .NET: The new
version includes an explicit method for releasing
a Gurobi license. You no longer need to rely on
the garbage collector to reclaim unused licenses.
* New methods, attributes, parameters, and error
codes: To support the new features in Gurobi 4.0,
we have added several new methods, attributes,
parameters, and error codes. You can learn more
about these in the {Gurobi Reference Manual}.
New methods:
* New C methods for managing Q: the C interface
includes three new routines, GRBaddqpterms,
GRBdelq, and GRBgetq. These allow you to add,
delete, and retrieve quadratic objective terms,
respectively.
* Quadratic expressions: the object oriented
interfaces include a new quadratic expression
class, GRBQuadExpr, which can be used to build
quadratic objective functions.
* getObjective/setObjective: the object oriented
interfaces include new GRBModel methods that allow
you to retrieve the current objective function as
a linear or quadratic expression, and allow you to
set the objective equal to a linear or quadratic
expression.
* getValue: the object oriented interfaces include a
new getValue method that allows you to compute the
value of a GRBLinExpr or GRBQuadExpr object for
the current solution.
* License release: the Java and .NET interfaces
include a new release method that allows you to
release the license held by an environment
immediately, instead of having to wait for the
garbage collector to reclaim the GRBEnv object.
New attributes:
* IsQP: model attribute that indicates whether the
model has any quadratic terms.
* NumQNZs: model attribute that returns the number
of quadratic terms in the objective function.
New parameters:
* Method: the previous LPMethod parameter has been
renamed to Method. This new parameter controls the
algorithm used to solve continuous linear and
quadratic models. We have added two new options:
concurrent and deterministic concurrent. This
parameter also selects the algorithm used to solve
the root node of a MIP model.
* NodeMethod: chooses the algorithm used to solve
node relaxations in a MIP model.
* ModKCuts: controls the generation of mod-k cuts.
* ImproveStartGap: allows you to specify the
optimality gap at which the MIP solver resets a
few MIP heuristics parameters in order to shift
the attention of the MIP solver to finding the
best possible feasible solution.
* ImproveStartTime: allows you to specify the
elapsed time at which the MIP solver resets a few
MIP heuristics parameters in order to shift the
attention of the MIP solver to finding the best
possible feasible solution.
* PreMIQPMethod: chooses the presolve transformation
performed on MIQP models.
* PSDTol: sets a limit on the amount of diagonal
perturbation that the optimizer is allowed to do
on the Q matrix for a quadratic model. If a larger
perturbation is required, the optimizer will
terminate with an GRB_ERROR_Q_NOT_PSD error.
New error codes:
* GRB_ERROR_Q_NOT_PSD: This new error code is
returned when you attempt to solve a QP model
where the Q matrix is not positive semi-definite
(meaning there exists an x for which x'Qx ). Note
that the optimizer will always try to add a small
perturbation to the diagonal to correct small PSD
violations. This error will be reported when the
required perturbation is too large (as controlled
by the new PSDTol parameter).
° ²° COMMENTS °² °
Do NOT distribute this release outside of the scene
Keep the scene alive and secure!
All good progs start as freeware,
then things get worse ...;-)
±²² ²²±
²²°² INSTALLATION NOTES ²°²²
±² ² `TLB' ² ²±
± ± Try it, Like it, Buy it! ± ±
° ° °
1. Unpack and install.
2. Copy the included files over the originals.
That's all. Have fun using it!;-)
___________________________________________________________________
Always remember to block applications (or go off line) from calling
home 'during install'. Once installed, disable 'check for automatic
updates' option if available, so that you don't get it blacklisted.

本資源帖謝絕任何廣告及與資源無關的評論,凡涉及廣告內容的評論一概刪除處理!
- 上一頁:《報稅軟件》(Intuit TurboTax Home and Business)v2010[壓縮包]
- 下一頁:《決策分析軟件》(TreeAge Pro Suite)v2011.1.0.12.1[壓縮包]
相關資源:
- [應用軟件]《漢王文本王文豪7600綠色簡潔專業版》(HWDOC-KING)[壓縮包]
- [安全相關軟件]《密碼恢復軟件》(L0phtCrack Password Auditor Enterprise)v6.0.12c企業版/含破解文件[壓縮包]
- [行業軟件]《工控》(Siemens.Simatic.WinCC.Web.Navigator.v6.2.SP2.MULTiLANGUAGE.ISO
- [應用軟件]《金山詞霸2005》(Power Word 2005)龍卷風會員版V2.0
- [軟件綜合]《veket系統QQ2013》(QQ2014 for veket)2013sp6[安裝包]
- [應用軟件]《遠程接入(瑞友天翼、2X、SecurityAdministration、citrix等)》4.0.0.0[壓縮包]
- [編程開發]《開發工具》(REALBasic Professional v2008 r5.1 Multilingual)[壓縮包],編程開發、資源下載
- [應用軟件]最新網吧破解任何收費端軟件
- [多媒體類]《音樂播放》(Cowon.JetAudio)v8.0.4.1000[壓縮包]
- [操作系統]《Tubro Linux 簡體中文版6.0》(Tubro Linux 6.0)簡體中文版6.0[ISO],操作系統、資源下載
- [少兒圖書]E 仁華學校 5-6年級奧數思維導引 上 學生版
- [生活圖書]《熟門熟路看中醫》掃描版[PDF]
- [游戲綜合]《瘋狂游戲大亨》免安裝簡體中文綠色版
- [英語學習資料]Bobby Rush -《Down in Louisiana》(在路易斯安那州)[FLAC] 音樂下載
- [其他圖書]《健身太極拳》掃描版[PDF]
- [英語學習資料]《ESLPod 英語播客課程(21世紀的新概念英語)-iPod慢速同步字幕版(新增第561課)》(ESL Podcast)【同步字幕有聲讀物,支持iPod、iPhone、iPad 4.2+、Android(暴風影音)、QuickTime】
- [藝術體育]《簡化吳式太極劍》北京電視藝術中心音像出版社[RMVB]
- [應用軟件]《Path Finder 資源管理器》(Path Finder)v5.6.6.1010 MacOSX Snow Leopard/破解版[壓縮包]
- [操作系統]《紅帽企業級Linux AS版》(Redhat Enterprise Linux AS v4.0 UPDate 7)[ISO]
- [編程開發]《aspnet學習包》(starter_Kits.zip),編程開發、資源下載
- 《Creo 3D插圖軟件》(PTC Creo Illustrate 1.0 F000 Win32/64)簡體中文(多國語言)版[光盤鏡像]
- 《服務器管理控制》(Mcafee Flashbox v4.6 VMware)VMware[光盤鏡像]
- 《建築設計軟件》(GraphiSoft ArchiCAD 13 International X86 )13 International X86[光盤鏡像]
- 《三維動畫制作軟件》(NewTek LightWave 3D)V11.5 Win32+Win64[壓縮包]
- 《建築三維渲染軟件》(Abvent.Artlantis.Studio)v3.0.2.1-REDT[壓縮包]
- 《賽捷薪資管理軟件》(Sage Payroll Solutions v12.01.029),行業軟件、資源下載
- 《Autodesk Inventor Professional 11 英文版》(Autodesk Inventor Professional 11)11[ISO],行業軟件、資源下載
- 《FIDES市政工程分析軟件套裝》(FIDES Pack)[壓縮包]
- 《地震目標檢測系統》(DGB OpendTect Commercial)v4.2.0b x86|x64[壓縮包]
- 《CAD/CAM/CAE集成軟件》(PTC Creo Elements/Pro 5.0 M090 Win64)簡體中文(多國語言)版[光盤鏡像]
- 《歐特克水暖電工程設計》(Autodesk Revit MEP 2012)WIN32/WIN64[光盤鏡像]
- 《PTC PRO ENGINEER WILDFIRECAD/CAM/CAE集成軟件》(PTC PRO ENGINEER WILDFIRE V4 M060)4.0.M060[光盤鏡像],行業軟件、資源下載
免責聲明:本網站內容收集於互聯網,本站不承擔任何由於內容的合法性及健康性所引起的爭議和法律責任。如果侵犯了你的權益,請通知我們,我們會及時刪除相關內容,謝謝合作! 聯系信箱:[email protected]
Copyright © 電驢下載基地 All Rights Reserved



