電驢下載基地 >> 图书资源 >> 教育科技 >> 《有限群的表示論與結合代數》(Representation Theory of Finite Groups and Associative Algebras )(Charles W. Curtis and Irving Reiner)清晰版[DJVU]
| 《有限群的表示論與結合代數》(Representation Theory of Finite Groups and Associative Algebras )(Charles W. Curtis and Irving Reiner)清晰版[DJVU] | |
|---|---|
| 下載分級 | 图书资源 |
| 資源類別 | 教育科技 |
| 發布時間 | 2017/7/10 |
| 大 小 | - |
《有限群的表示論與結合代數》(Representation Theory of Finite Groups and Associative Algebras )(Charles W. Curtis and Irving Reiner)清晰版[DJVU] 簡介: 中文名 : 有限群的表示論與結合代數 原名 : Representation Theory of Finite Groups and Associative Algebras 作者 : Charles W. Curtis and Irving Reiner 資源格式 : DJVU 版本 : 清晰版 出版社 : American Mathematical Society 書號
電驢資源下載/磁力鏈接資源下載:
全選
"《有限群的表示論與結合代數》(Representation Theory of Finite Groups and Associative Algebras )(Charles W. Curtis and Irving Reiner)清晰版[DJVU]"介紹
中文名: 有限群的表示論與結合代數
原名: Representation Theory of Finite Groups and Associative Algebras
作者: Charles W. Curtis and Irving Reiner
資源格式: DJVU
版本: 清晰版
出版社: American Mathematical Society
書號: 0821840665
發行時間: 2006年
地區: 美國
語言: 英文
簡介:

內容簡介:
本書概述了有限群,結合環及結合代數表示論。除了Burnside書中表示論的經典內容,本書著重敘述了誘導特征,誘導表示,擬Frobenius環,Frobenius代數,整數表示及模表示論.迄今為止。其中的許多內容僅見於研究論文中。本書注重一般方法,將理論構建於滿足極小條件環上的模。為幫助研究者得到特定群的具體表示,書中包含了大量的例子以及問題,並給出了一些群表示應用於有限群結構理論的例子。
本書前三章給出了一些介紹性的材料,同時也為後續的章節提供了背景.
4-7章介紹了滿足極小條件的半單環的結構理論及其在群表示與特征上的應用
4,8,9,10章介紹了滿足極小條件的環及有限維代數
3,11章介紹了代數數論及群的整數表示
12章介紹了模表示論
First published in 1962, this classic book remains a remarkably complete introduction to various aspects of the representation theory of finite groups. One of its main advantages is that the authors went far beyond the standard elementary representation theory, including a masterly treatment of topics such as general non-commutative algebras, Frobenius algebras, representations over non-algebraically closed fields and fields of non-zero characteristic, and integral representations. These and many other subjects are treated extremely thoroughly, starting with basic definitions and results and proceeding to many important and crucial developments. Numerous examples and exercises help the reader of this unsurpassed book to master this important area of mathematics.
內容截圖:
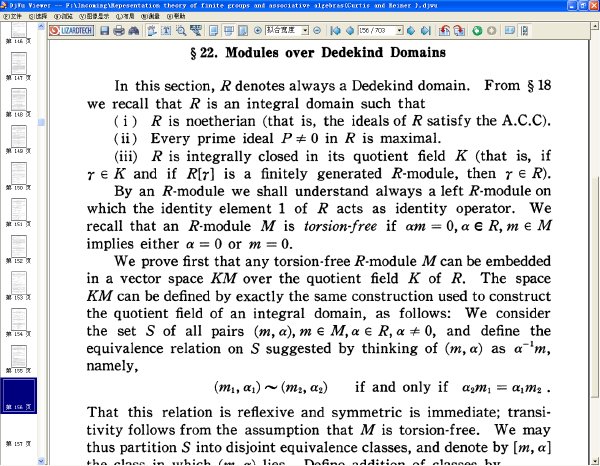
目錄:
Notation
I.Background from Group Theory
II.Representations and Modules
III.Algebraic Number Theory
IV.Semi-simple Rings and Group Algebras
V.Group Characters
VI.Induced Characters
VII.Induced Representations
VIII.Non-Semi-Simple Rings
IX.Frobenius Algebras
X.Splitting Fields and Separable Algebras
XI.Integral Representations
XII.Modular Representations
Index
原名: Representation Theory of Finite Groups and Associative Algebras
作者: Charles W. Curtis and Irving Reiner
資源格式: DJVU
版本: 清晰版
出版社: American Mathematical Society
書號: 0821840665
發行時間: 2006年
地區: 美國
語言: 英文
簡介:

內容簡介:
本書概述了有限群,結合環及結合代數表示論。除了Burnside書中表示論的經典內容,本書著重敘述了誘導特征,誘導表示,擬Frobenius環,Frobenius代數,整數表示及模表示論.迄今為止。其中的許多內容僅見於研究論文中。本書注重一般方法,將理論構建於滿足極小條件環上的模。為幫助研究者得到特定群的具體表示,書中包含了大量的例子以及問題,並給出了一些群表示應用於有限群結構理論的例子。
本書前三章給出了一些介紹性的材料,同時也為後續的章節提供了背景.
4-7章介紹了滿足極小條件的半單環的結構理論及其在群表示與特征上的應用
4,8,9,10章介紹了滿足極小條件的環及有限維代數
3,11章介紹了代數數論及群的整數表示
12章介紹了模表示論
First published in 1962, this classic book remains a remarkably complete introduction to various aspects of the representation theory of finite groups. One of its main advantages is that the authors went far beyond the standard elementary representation theory, including a masterly treatment of topics such as general non-commutative algebras, Frobenius algebras, representations over non-algebraically closed fields and fields of non-zero characteristic, and integral representations. These and many other subjects are treated extremely thoroughly, starting with basic definitions and results and proceeding to many important and crucial developments. Numerous examples and exercises help the reader of this unsurpassed book to master this important area of mathematics.
內容截圖:

目錄:
Notation
I.Background from Group Theory
II.Representations and Modules
III.Algebraic Number Theory
IV.Semi-simple Rings and Group Algebras
V.Group Characters
VI.Induced Characters
VII.Induced Representations
VIII.Non-Semi-Simple Rings
IX.Frobenius Algebras
X.Splitting Fields and Separable Algebras
XI.Integral Representations
XII.Modular Representations
Index
- 上一頁:《相交理論》(Intersection Theory)掃描版[DJVU]
- 下一頁:《人格心理學:了解他人(第四版)》(Theories of Personality:Understanding Persons (4th Edition))掃描版[PDF]
相關資源:
- [生活圖書]《實戰摔法招招絕》掃描版[PDF]
- [人文社科]《民國風雲秘錄》掃描版[PDF]
- [教育科技]《國際商務洽談口語教程》掃描版[PDF]
- [文學圖書]《傾城之戀》(張愛玲)影印版[PDF]
- [人文社科]《真實的幸福》(Authentic Happiness)掃描版[PDF]
- [計算機與網絡]《IT安全面試攻略》(IT Security Interviews Exposed: Secrets to Landing Your Next Information Security Job)掃描版[PDF]
- [其他圖書]《《讀者參考》叢書:廣匯博集》(《讀者參考》編輯部)掃描版[PDF]
- [經濟管理]《簡單學 在最短的時間內,以最小的付出獲得你想要的》掃描版[PDF]
- [人文社科]《害羞心理學》掃描版[PDF]
- [生活圖書]《旅行攝影大師班》掃描版[PDF]
- [人文社科]《英雄連2》(Company of Heroes 2)完整硬盤版 + 2號升級破解補丁 + 3DM漢化補丁V4
- [多媒體類]《Corel 藍光和DVD播放器軟件》(Corel WinDVD Pro 2010)v10.0.5.713 Multilingual[壓縮包]
- [學習課件]《周易譯注》(黃壽祺 & 張善文)2007年修訂版[PDF] 資料下載
- [生活圖書]《攝影月刊
- [光盤游戲]《情聖拉瑞:優等生》(Leisure.Suit.Larry.Magna.Cum.Laude)[Bin]
- [經濟管理]《一課之師》(亨利.黑茲利特)掃描版[PDF]
- [安全相關軟件]《文件夾加密工具》(Kruptos 2 Professional)v3.0.0.15 x86|x64[壓縮包]
- [編程開發]《CJC Delphi百事通》(CJC Delphi)1.50
- [教育綜合]蘇教版小學語文配套光盤一年級 上冊
- [游戲綜合]《問情OL》V1.0179 不刪檔內測客戶端
免責聲明:本網站內容收集於互聯網,本站不承擔任何由於內容的合法性及健康性所引起的爭議和法律責任。如果侵犯了你的權益,請通知我們,我們會及時刪除相關內容,謝謝合作! 聯系信箱:[email protected]
Copyright © 電驢下載基地 All Rights Reserved



